I have taken the dataset from pentacene CSP (supplementary data from J. E. Campbell, J. Yang and G. M. DayPredicted energy–structure–function maps for the evaluation of small molecule organic semiconductors). Together 586 structures. Next I have computed minimum distance  (2-norm) between pentacene molecules within every structure (0.5573). (The van der Walls radii are not good because some pentacene molecules in the structures overlap when the balls have this radius). The length of the covalent bond between two adjacent carbons is 1.3618 Angstroms.  Based on this I have created a point cloud with 1108 points on a sphere around every atom of pentacene with the radius 0.5573/2.

Below are the visualizations of convex hulls of the point cloud for 2 structures. On the left is the structure where the mentioned minimum is attained.

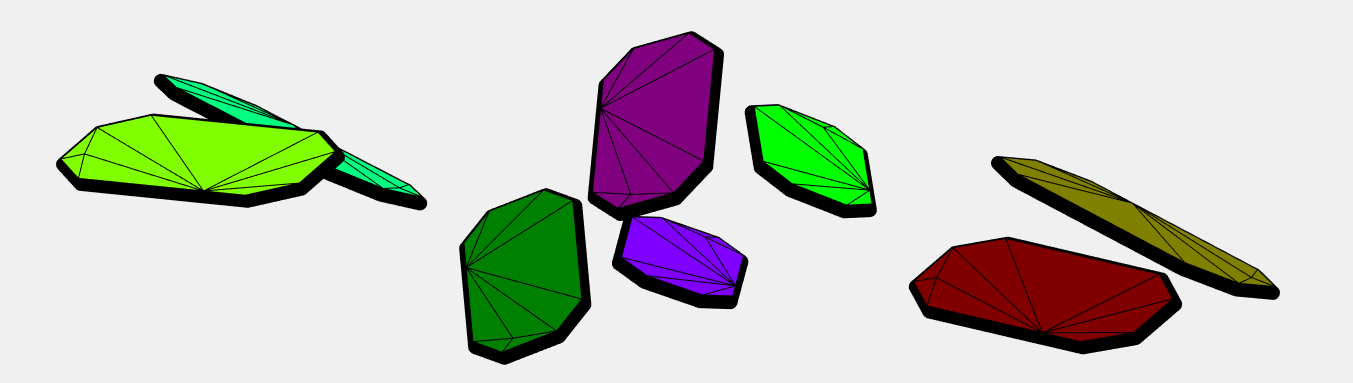
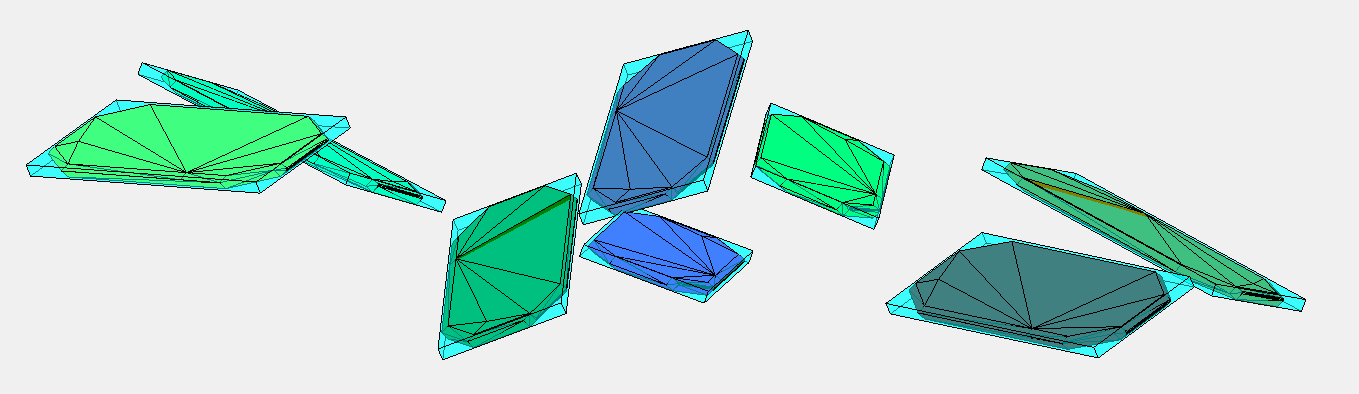
The O’Rourke’s minimum bounding box approach is not bad either, but we have to make the point could radius even smaller.

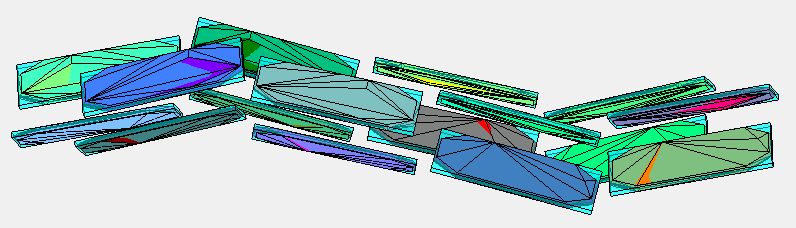
Conclusion: I think we should work with convex hulls.

Comment 1. : I discovered a stupid error I made with the minimal enclosing boxes modeling. Here it’s corrected but all the previous ones are most likely wrong.

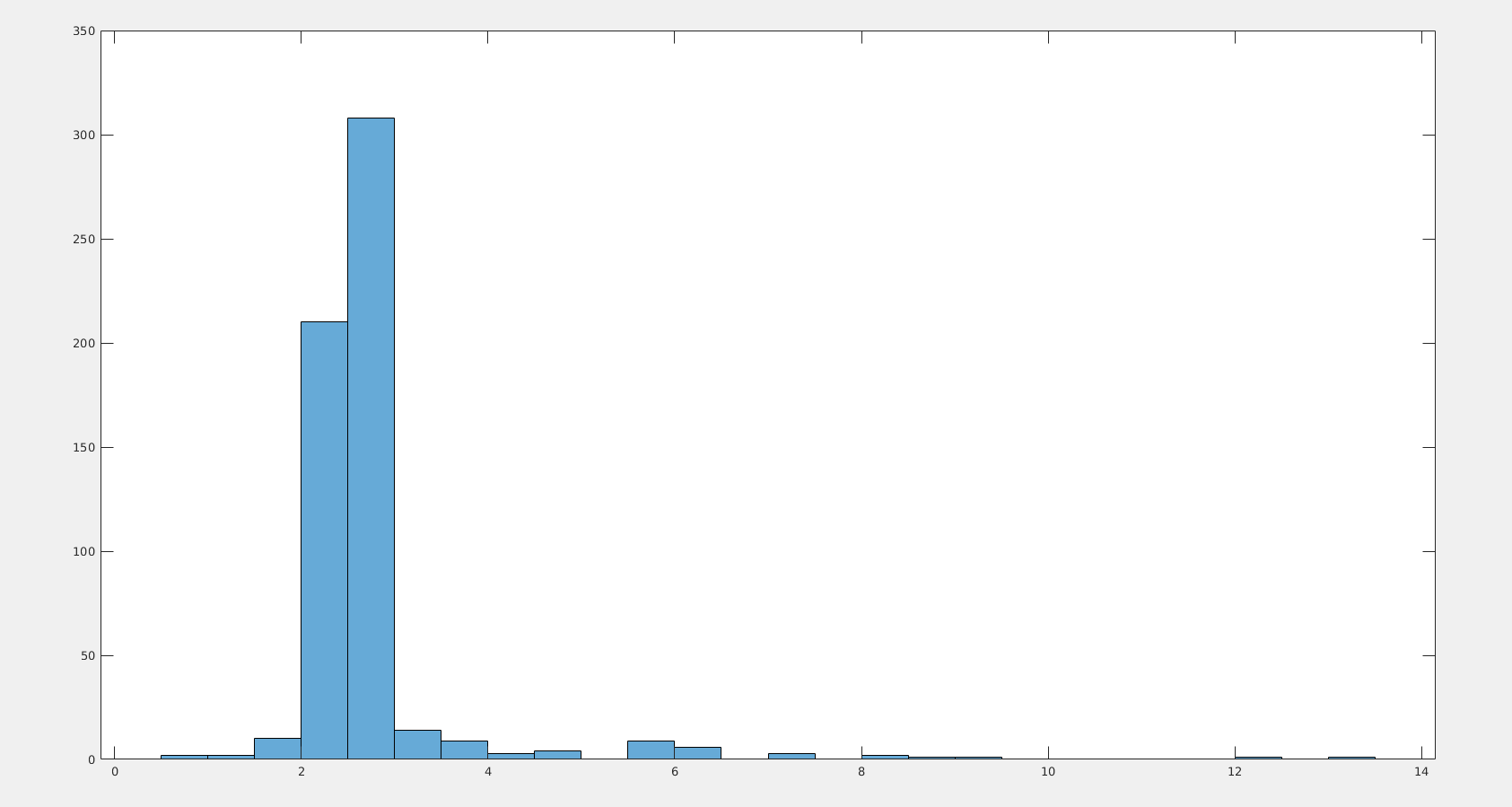
Comment 2. : the median of the minimum distances between pentacene molecules within the 586 structures is 2.6197. The histogram of the minima is below.

I applied the minimum bounding box to another clustering of the cage 3 molecule as in previous cases

, to all clusters of the cage 3 molecule

and to the whole cage 3 crystal

Conclusion: Few of the boxes of different cage 3 molecules overlap a bit.

I applied the minimum bounding box to the two joined asymmetric units of the cage 3 molecule

, to all joined asymmetric units of the cage 3 molecule

and to the cage 3 crystal

Conclusion: the boxes overlap.

I applied the minimum bounding box to the asymmetric unit of the cage 3 molecule

,to all asymmetric units of the cage 3 molecule

and to the cage 3 crystal

Conclusion: The boxes of different cage 3 molecules still overlap.

I played a bit with the alpha shapes of the centroids of the carbon clusters in Cage 3 molecule.

Just a visualization of how  the cubes could be arranged.

Initial setup

Possible shifts

 

A visualization of how good of a model the minimum enclosing box is when applied to a real crystal structure.

Alpha shape applied to the Cage 3 molecule in the minimum enclosing box

Alpha shapes in a Cage 3 crystal

The alpha shapes do not overlap.  For some alpha.

Convex hulls in a Cage 3 crystal

The convex hulls of Cage 3 overlap.

Enclosing boxes in a Cage 3 crystal

 

I started work with only with one enclosing box and then to continue to build upon that.

I took one enclosing box and constructed a unit cell by surrounding this central box by other boxes (as is visualized in previous post). Next I “optimized” lattice energy by Lennard-Jones potential using the centers of mass of the CC3 molecule. The central cube can by moved in 3-directions (x,y,z) but out of symmetry it’s sufficient to move only in direction in this particular case. The center of mass of CC3 is slightly off the center of mass of the small cube. That means there are 16 rotations of the central cube that can be potential minima of the energy function.

The result can by seen by below. The dots are centers of mass of CC3. The minimum was attained with no rotation of the central small box.

Slides from the presentation: lattice_packings.pdf

Minimal enclosing box computed via. O’Rourk’s algorithm. The box is actually a cube:

27 of these boxes stacked together forming a unit cell: