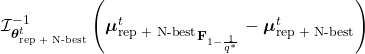Following up on the packing of the two-dimensional hexagonal molecules in the post Chiral Interaction Energy Ground States, from the materials science perspective the primary interest is in the three-dimensional molecular packings. So, the natural next step is to extend the insights gained by studying the symmetries of two-dimensional models of molecules as well as ionic molecular crystals outlined in a series of post:
Chiral Interaction Energy Ground States
Ionic Molecular Crystallization
Ionic Molecular Crystallisation Continued: Tetrahedra, Symmetry, and Salt-Like Molecular Architectures
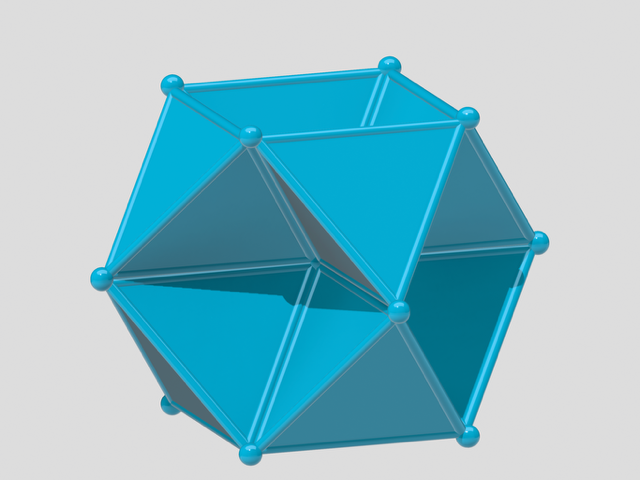
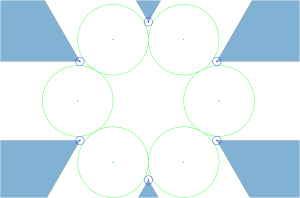
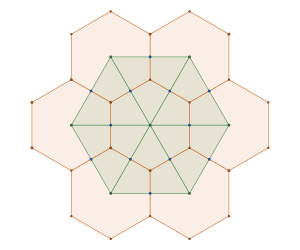
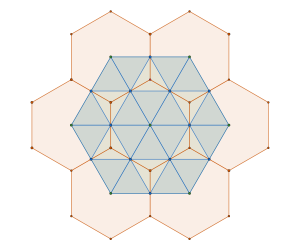
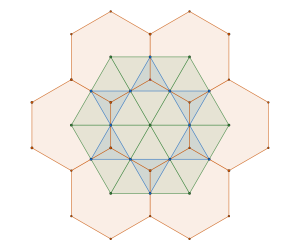
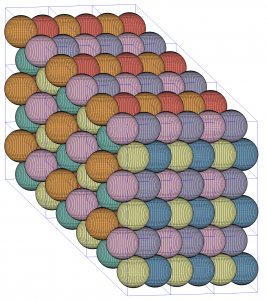
The hexagon is a radially equilateral 2-polytope. The equivalent 3-polytope is the Cuboctahedron; thus we will work with the representation of a molecule with cuboctahedral symmetry using a rigid hard sphere model (a molecule is a rigid collection of hard spheres) as visualised in the figures below.



Problem Statement
We are interested in the following question: What is the densest packing of a hard sphere molecule model with cuboctahedral symmetry?
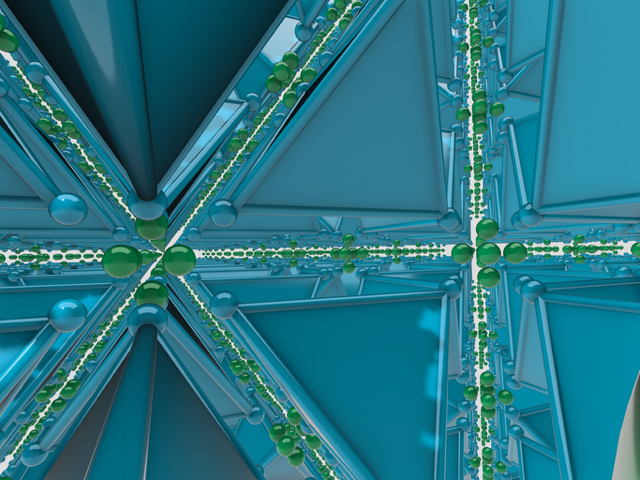
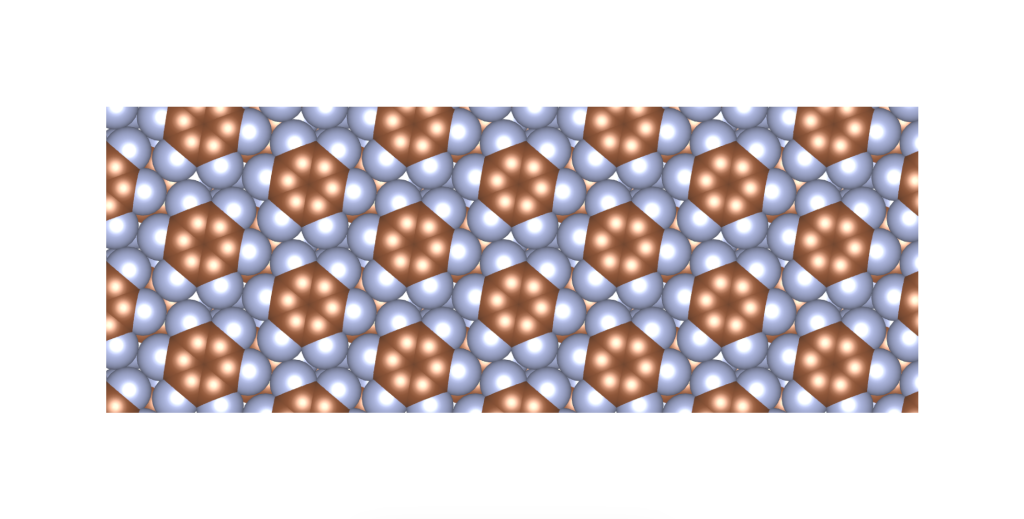
The GEOMAG structure shown in the animation below is a good candidate. It has layers of hexagonal packings just like the perfluorobenzene structure in Chiral Interaction Energy Ground States but is it truly the densest possible configuration? I’ll leave you wondering for a bit longer (or you skip to the conclusion of the quacked case in section 6 at the end of this post).
Divide and Conquer
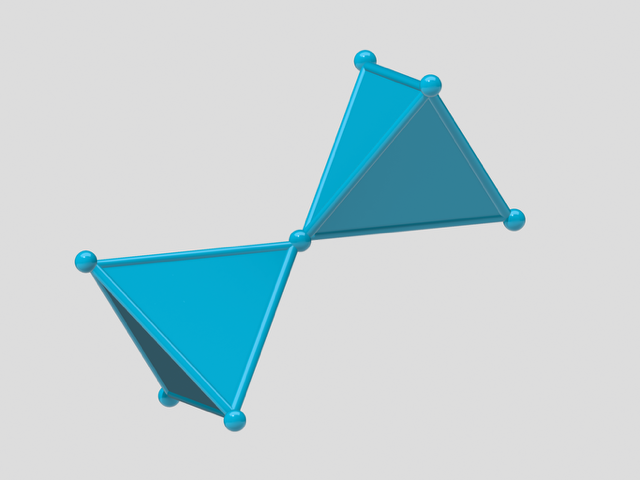
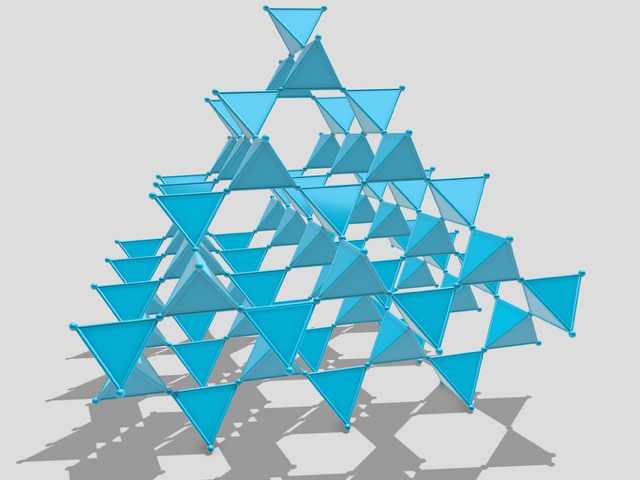
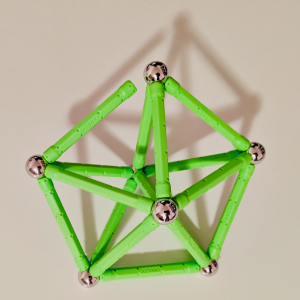
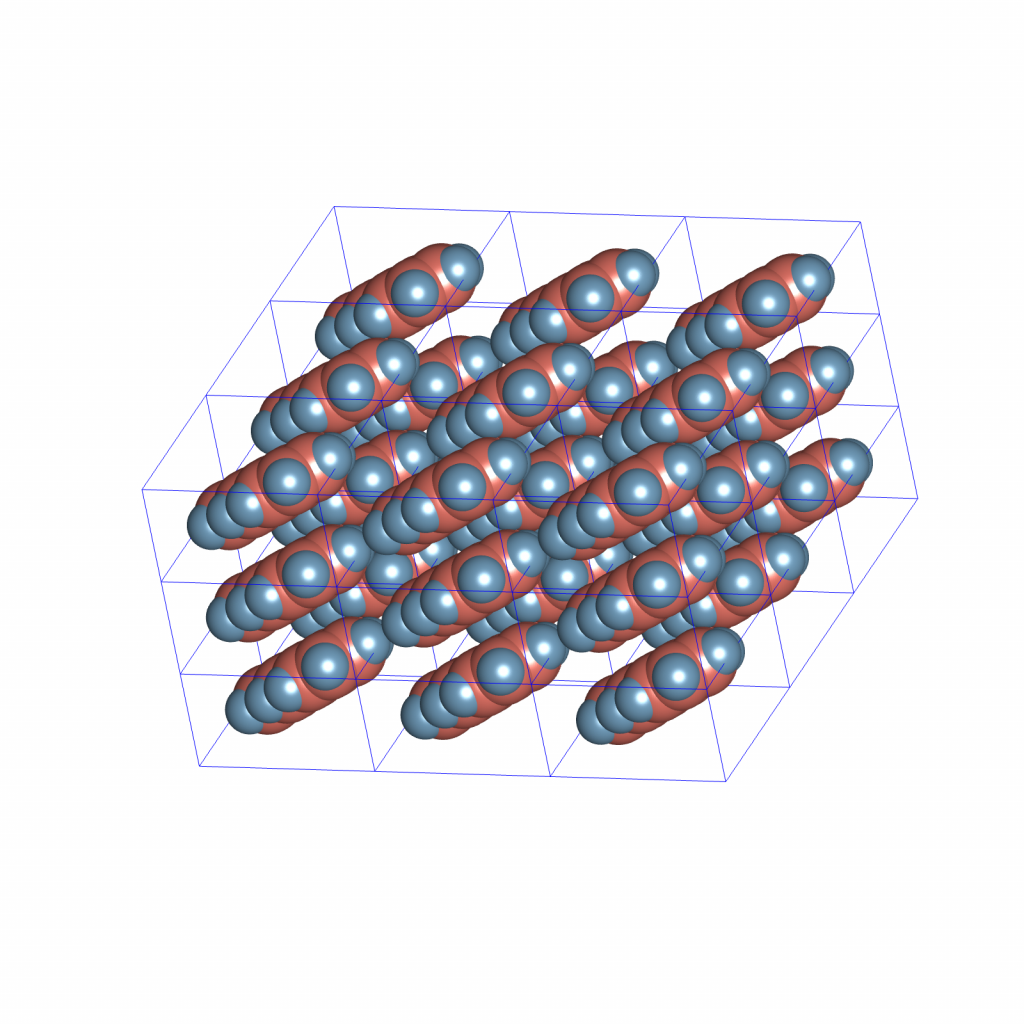
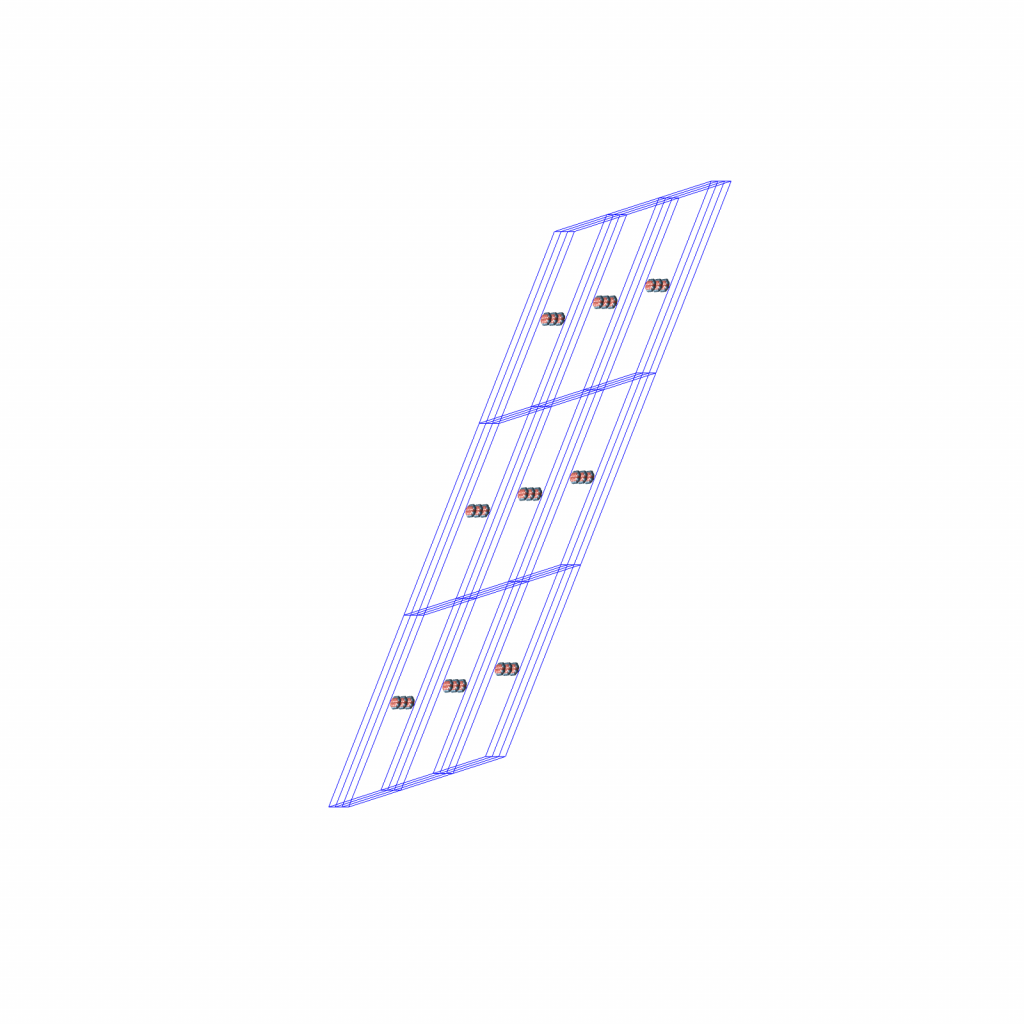
In the spirit of the divide and conquer problem solving strategy, it might be worthwhile to start with packings of simpler model molecules. Specifically, molecules having tetrahedral and octahedral symmetries like the GEOMAG constructions below, since the tetrahedron and octahedron are related to the cuboctahedron via the tetrahedral-octahedral honeycomb.

How do we know this is the optimal packing configuration of tetrahedral molecules? Well, by definition, a tetrahedral molecule is a collection of equal spheres with radius of half of the minimum distance between any two atoms of the molecule. Thus every tetrahedral molecule is a sphere packing of four spheres with equal radii. Additionally, since the kissing number of a 3-sphere is 12 (every sphere can touch a maximum of 12 neighbouring vertices) and every vertex of the GEOMAG structure belongs to exactly one tetrahedron the density of packing of tetrahedral molecules equals the Face-Center Cubic (FCC) close-packing of equal spheres, and we know we can’t get any denser packing then this due to the proof of Kepler conjecture by Thomas Hales.
The optimality of the GEOMAG octahedral molecule crystal model follows directly from the optimality of the tetrahedral variant.
Let us numerically proof-check our results using our packing algorithm outlined in LRC Symposium Supplementary Materials LRC Symposium Supplementary Materials. Here we are presented with another problem. Out of 230 space groups listed IUCr International Tables of Crystallography, which space group should we start searching first?
Closest-Packed Space Groups
From the GEOMAG constructions, we can see that there are at least two kinds of symmetries present:
1. Lattice translations – tetrahedra with the same colour are related by this isometry. The configuration of octahedral molecule models is in fact a lattice configuration. That is, the octahedral structure is generated only by a lattice group.
2. Inversions – tetrahedra and octahedra of different colours are related by this isometry. Since the regular octahedron is centrosymmetric, one can view the octahedra of different colours as inversions of each other. This introduces the inversion symmetry to the whole octahedral structure.
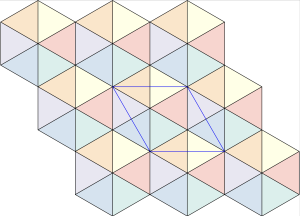
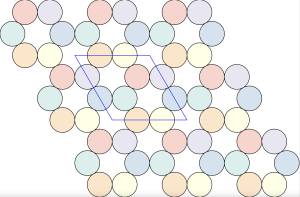
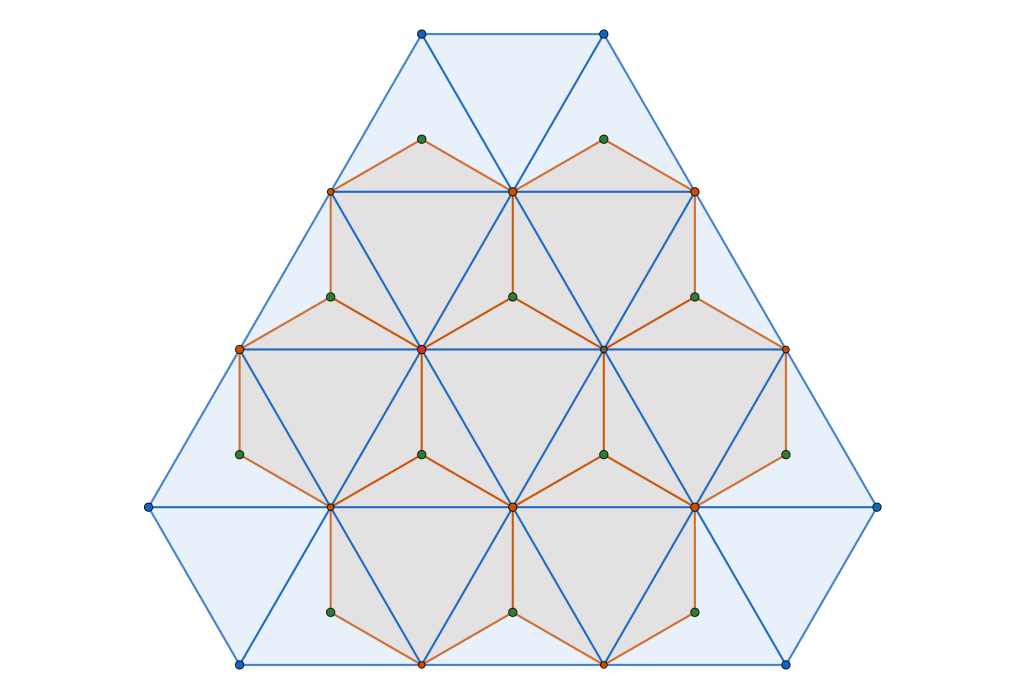
The lowest symmetry space group containing these isometries is the space group ![]() . See the densest packing configuration found during one optimisation run of the molecular packing algorithm’s search in the space group
. See the densest packing configuration found during one optimisation run of the molecular packing algorithm’s search in the space group ![]() .
.



The volume of the unit cell of this structure is ![]() . Since the volume of our tetrahedral molecule is
. Since the volume of our tetrahedral molecule is ![]() (4 times the volume of a sphere with radius
(4 times the volume of a sphere with radius ![]() ), according to the geometric packing density formula
), according to the geometric packing density formula ![]() and the number of elements of factor group
and the number of elements of factor group ![]() where
where ![]() is the lattice subgroup of
is the lattice subgroup of ![]() , is
, is ![]() ,
, ![]() which is exactly the density of FCC close-packing of equal spheres.
which is exactly the density of FCC close-packing of equal spheres.



Crystallographers like to assign a space group name to a structure according to its highest symmetry. The question is, what is the highest symmetry of our densest tetrahedral molecular packing? To answer this question, we have to do a quick excursion into topology, specifically “orbit-manifolds” or, for short, orbifolds.
Fibrifold Primer
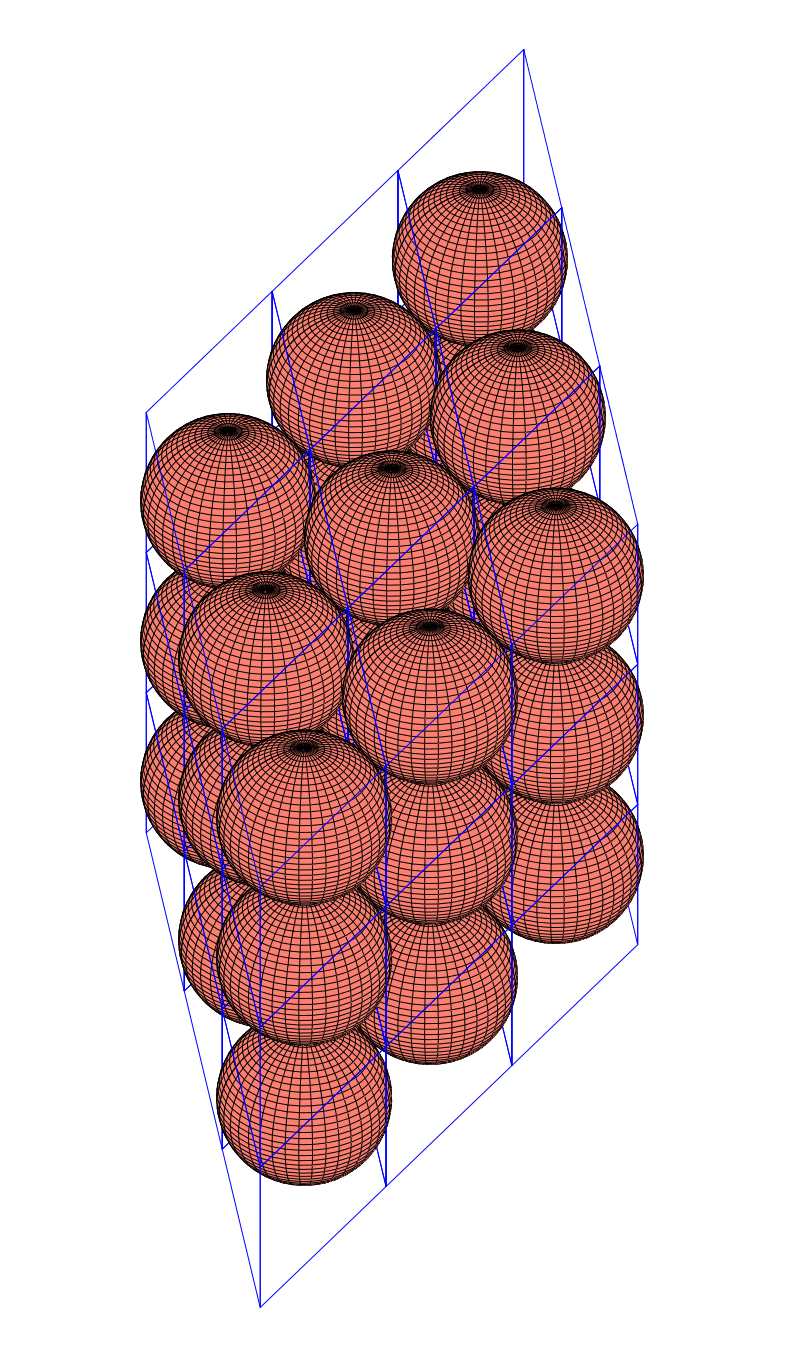
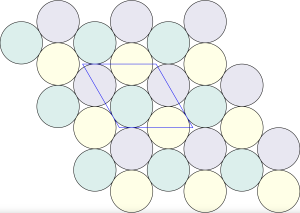
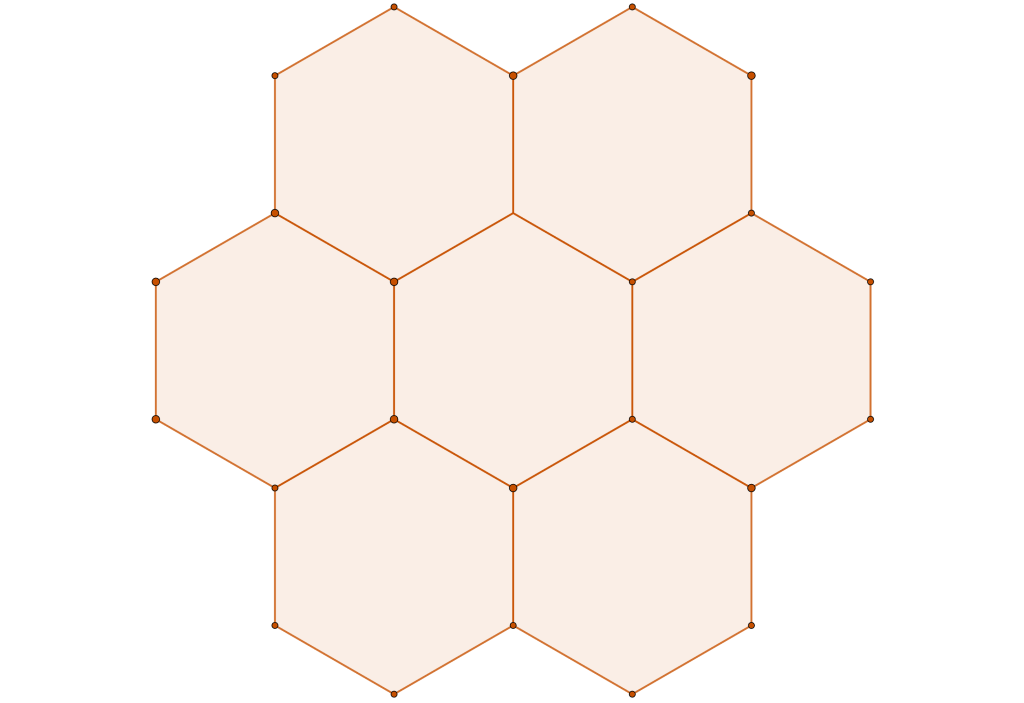
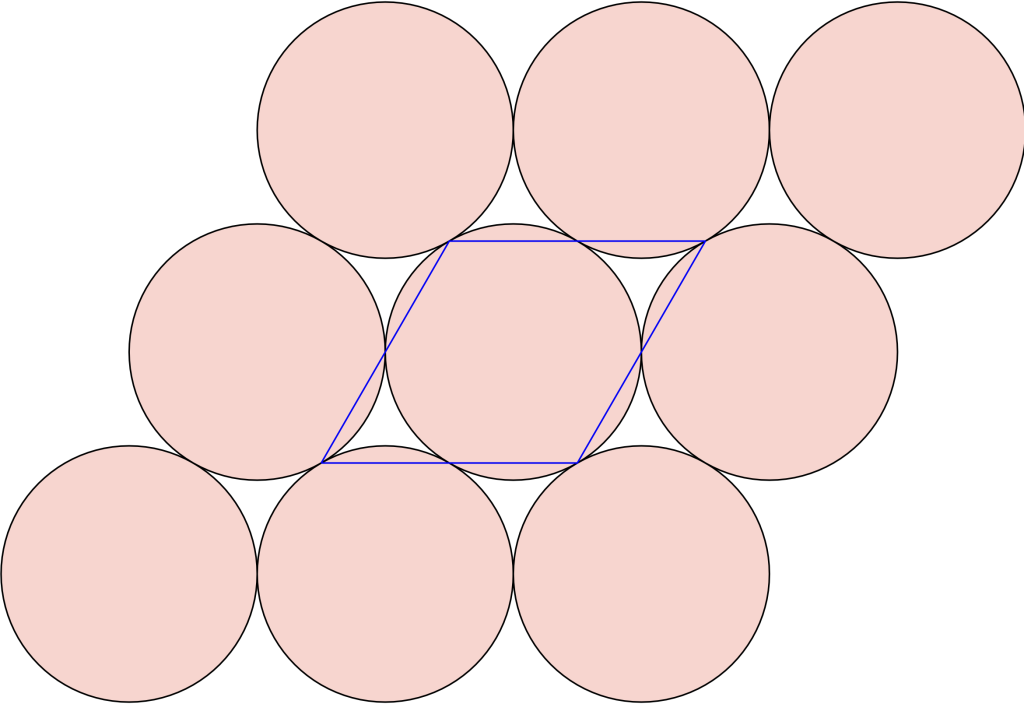
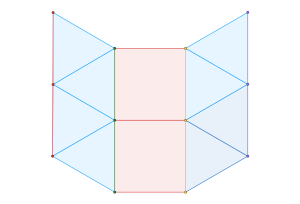
Let us take the vertices of a triangular lattice disc packing’s unit cell as highlighted by the blue parallelpiped in the image on the left below.


All four vertices are in fact centres of a 2-fold rotational symmetry as shown in the picture above on the right, making up the ![]() wallpaper group. Now if we fold up the this group by identifying the symmetry elements we end up with a surface akin to a sphere containing four singular points – points where the tangent space to the surface is not defined regularly.
This is the orbit-manifold, for short orbifolds, representation of the crystallographic plane group
wallpaper group. Now if we fold up the this group by identifying the symmetry elements we end up with a surface akin to a sphere containing four singular points – points where the tangent space to the surface is not defined regularly.
This is the orbit-manifold, for short orbifolds, representation of the crystallographic plane group ![]() . The following figure, taken from the book The Symmetries of Things by John H. Conway, Heidi Burgiel, and Chaim Goodman-Strauss, illustrates this nicely
. The following figure, taken from the book The Symmetries of Things by John H. Conway, Heidi Burgiel, and Chaim Goodman-Strauss, illustrates this nicely

Since we are working with space groups we need to extend wallpaper orbifolds to space groups. In essence we need to add one more lattice generator which is equivalent to multiplying (in the sense of the Cartesian product) the ![]() orbifold with a circle and thus creating a “fibered orbifold” or a fibrifold.
orbifold with a circle and thus creating a “fibered orbifold” or a fibrifold.
Geometrically a fibrifold is a fibre-bundle whose base spaces and fibres are orbifolds. In the case of space group ![]() the fibre spaces are circles
the fibre spaces are circles ![]() . The primary fibrifold name of space group
. The primary fibrifold name of space group ![]() is
is ![]() .
.
Listing the Closest-Packed Symmetries
In the ![]() tetrahedral molecule packings we can notice interchanging layers of blue and red tetrahedral molecules stacked on top of each other. This is not a coincidence, these are the
tetrahedral molecule packings we can notice interchanging layers of blue and red tetrahedral molecules stacked on top of each other. This is not a coincidence, these are the ![]() orbifolds of the
orbifolds of the ![]() fibrifold coupled together. There are at most three such non-isomorphic orbifold couplings in every space group. That is why some fibrifolds have a secondary or tertiary name. In the IUCr International Tables of Crystallography they are called Special Projections.
fibrifold coupled together. There are at most three such non-isomorphic orbifold couplings in every space group. That is why some fibrifolds have a secondary or tertiary name. In the IUCr International Tables of Crystallography they are called Special Projections.
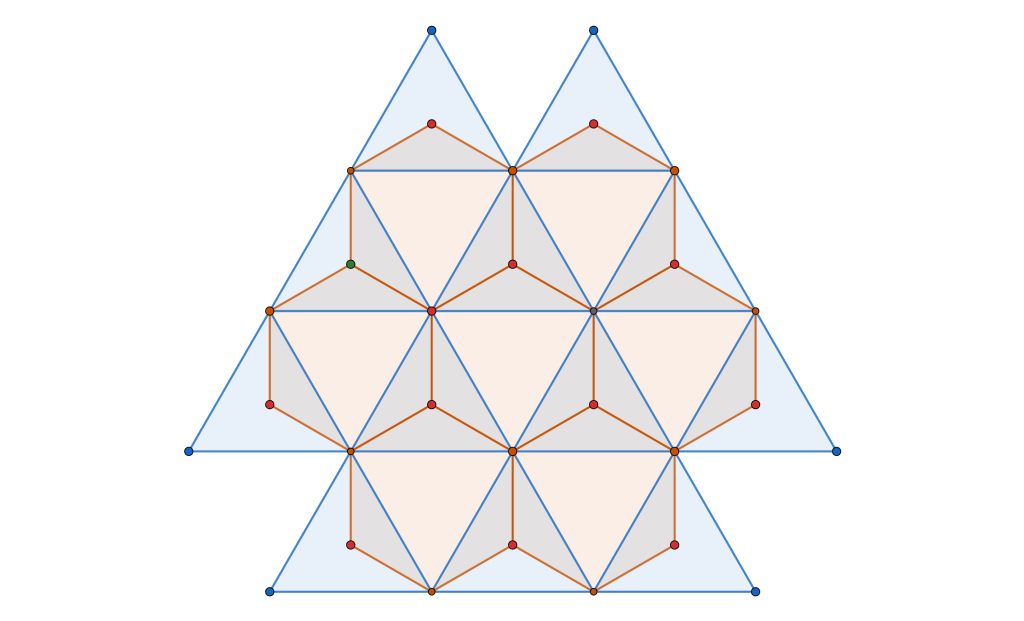
Below are these projections for the ![]() tetrahedral molecule packing.
tetrahedral molecule packing.







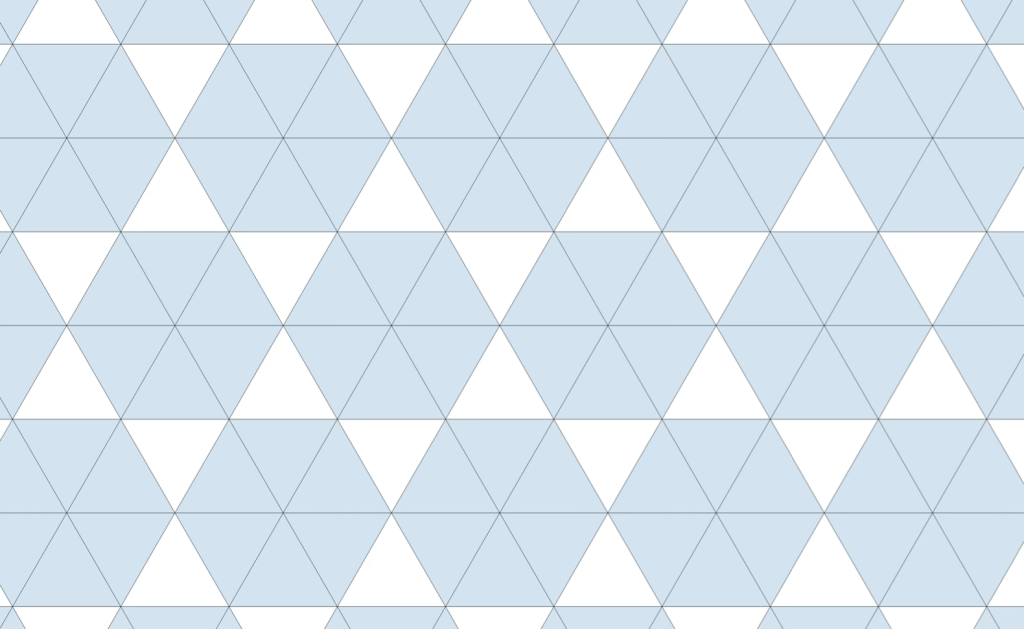
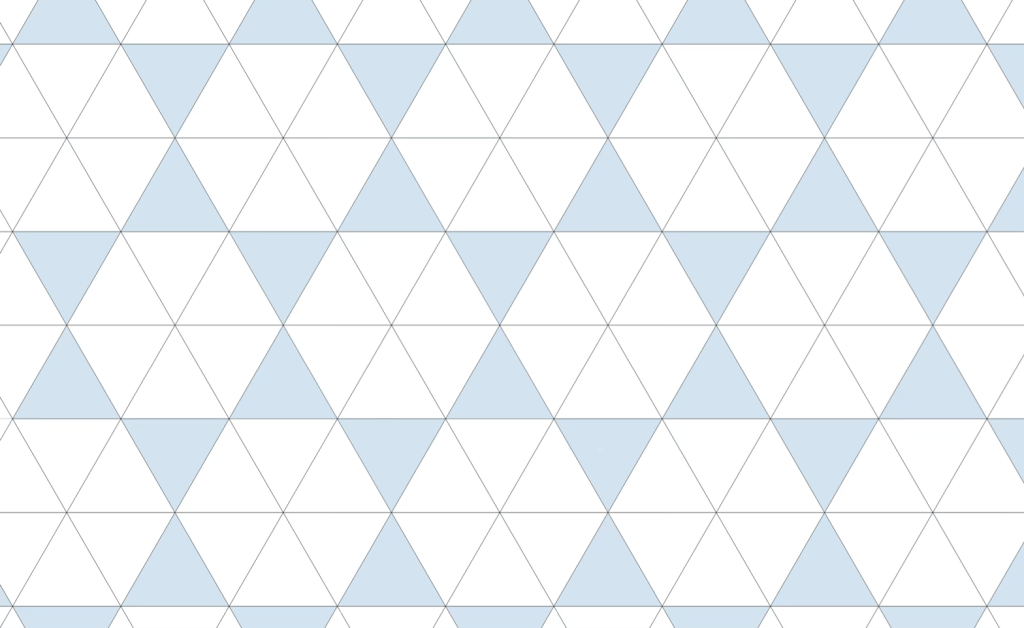
In this particular case the symmetries of special projections are all ![]() orbifolds i.e. the wallpaper group
orbifolds i.e. the wallpaper group ![]() . These projections are reminiscent of the triangle and square tilings from our analysis of the densest plane group packings of regular convex n-gons published in Physical Review E we know that all regular n-gons attain the maximum packing density in wallpaper groups
. These projections are reminiscent of the triangle and square tilings from our analysis of the densest plane group packings of regular convex n-gons published in Physical Review E we know that all regular n-gons attain the maximum packing density in wallpaper groups ![]() ,
, ![]() and
and ![]() with orbifold names
with orbifold names ![]() ,
, ![]() and
and ![]() respectively.
respectively.
 |
| 


 |
| 


 |
| 


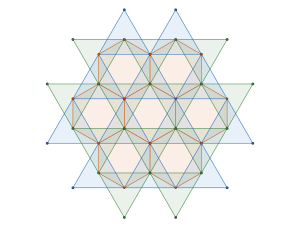
There are only three space groups having special projections a combination of these three orbifolds. These space groups are (the space group we started with in the first place), ![]() with the fibrifold name
with the fibrifold name ![]() and
and ![]() with the fibrifold name
with the fibrifold name ![]() .
.
Are these the only symmetries of the tetrahedral molecular packing? No, all three ![]() special projections contain a mirror reflection. Therefore we should further our space group explorations including the wallpaper group
special projections contain a mirror reflection. Therefore we should further our space group explorations including the wallpaper group ![]() (orbifold name
(orbifold name ![]() ), reflected in the
), reflected in the ![]() triangle and square tilings:
triangle and square tilings:
 |
| 


Thus we are adding the space groups ![]() with its fibrifold name
with its fibrifold name ![]() and
and ![]() with its fibrifold name
with its fibrifold name ![]() to our list.
to our list.
Are we done here? Not yet, one more step is necessary. Notice the ![]() (orbifold name
(orbifold name ![]() ) square tiling symmetry in one of the tetrahedral molecular packing special projections.
) square tiling symmetry in one of the tetrahedral molecular packing special projections.
 |
| 


For this reason the last space group to add to our list is ![]() . Its fibrifold name is
. Its fibrifold name is ![]() .
.
All the space groups in our closest-packed list are summarised in the table below.
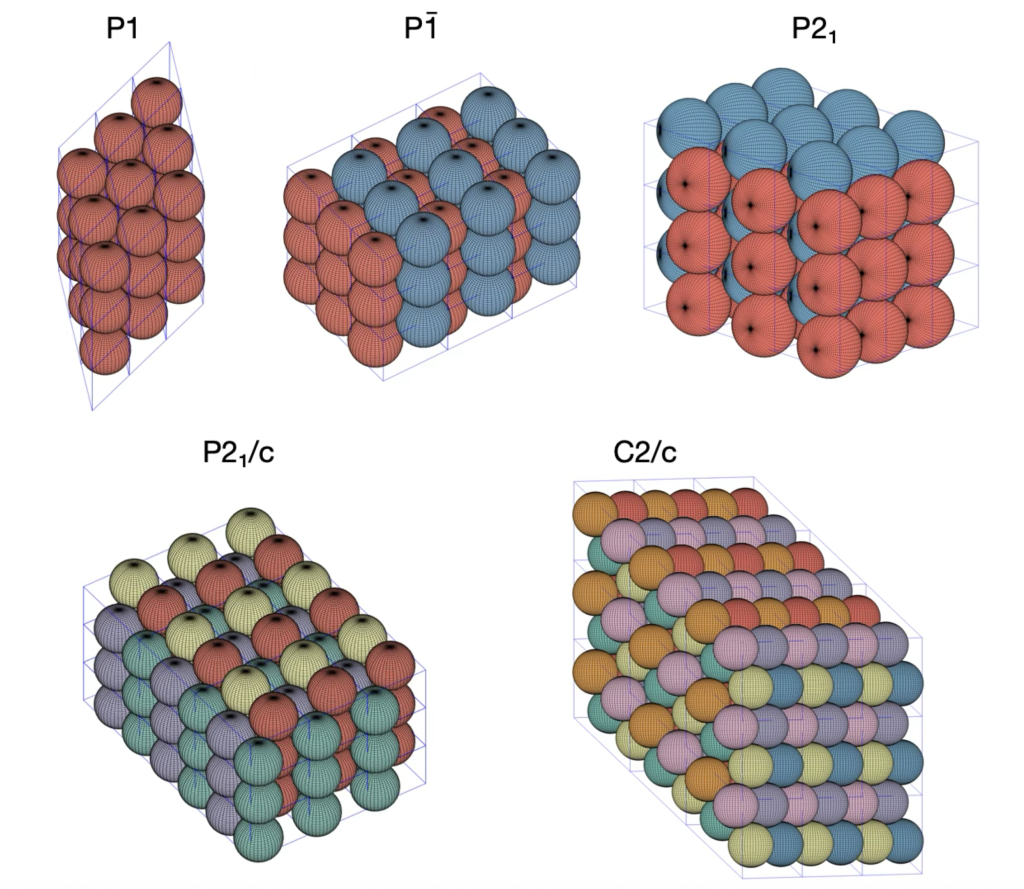
![Rendered by QuickLaTeX.com \[ \begin{tabular}{ll|ll|ll|ll} \hline \hline \multicolumn{8}{c}{\textbf{Symmetries of the} $\mathbf{P\bar{1}}$ \textbf{Tetrahedral and Octahedral Molecule Packings}} \\ \hline \multicolumn{2}{c|}{\textbf{Closest-Packed Space Groups}} & \multicolumn{6}{c}{\textbf{Symmetries of Special Projections}} \\ \hline \textit{H-M} & \textit{Fibrifold (primary)} & \textit{H-M} & \textit{Orbifold} & \textit{H-M} & \textit{Orbifold} & \textit{H-M} & \textit{Orbifold} \\ \hline $P\overline{1}$ & (2 2 2 2) & $p2$ & 2 2 2 2 & $p2$ & 2 2 2 2 & $p2$ & 2 2 2 2 \\ $P2_{1}$ & ($2_{1} 2_{1} 2_{1} 2_{1}$) & $pg$ & $\times \times$ & $pg$ & $\times \times$ & $p2$ & 2 2 2 2 \\ $P2_{1}/c$ & ($2_{1} 2_{1} 2 2$) & $p2mg$ & 2 2 * & $p2gg$ & 2 2 $\times$ & $p2$ & 2 2 2 2 \\ $C2/c$ & ($2_{0} 2_{1} 2 2$) & $c2mm$ & 2*2 2 & $p2mg$ & 2 2 * & $p2$ & 2 2 2 2 \\ $P2_{1}2_{1}2_{1}$ & ($2_{1} 2_{1} \overline{2}$) & $p2gg$ & 2 2 $\times$ & $p2gg$ & 2 2 $\times$ & $p2gg$ & 2 2 $\times$ \\ $Pbca$ & ($2_{1} 2\overline{*} :$) & $p2mg$ & 2 2 * & $p2mg$ & 2 2 * & $p2mg$ & 2 2 * \\ \hline \end{tabular} \]](https://milotorda.net/wp-content/ql-cache/quicklatex.com-7feaf7092df8b1622f700599e7a496b2_l3.png)
Navigating Singularities in Space Groups
Running searches over this space groups using our molecular packings algorithm required new a definition of an asymmetric unit in the packing algorithm for each space group such that all singular point sets of fibrifolds are contained on the boundary of the asymmetric unit. Unlike the definitions from the IUCr International Tables of Crystallography that we used before.
I wonder if companies developing software used in Crystal Structure Prediction workflows know about this unfortunate choice of asymmetric units in the IUCr International Tables of Crystallography because they also use second order numerical optimisation methods over space groups in local structure refinements and inevitably must encounter the same problem we did. Fortunately for us, equipped with the fibrifold perspective of space groups, we manage to avoid the singularities safely.
Densest  and
and  Tetrahedral Molecule Packings
Tetrahedral Molecule Packings
For the visualisation and a brief analysis of the output configuration in the space group ![]() search, we refer to section Closest-Packed Space Groups.
search, we refer to section Closest-Packed Space Groups.
As for the rest, by choosing to run searches in six very special space groups, the packing densities of all the densest molecular space group configurations with tetrahedral symmetry have the same maximal sphere packing density. The highest symmetry of the tetrahedral molecule packing in our list is ![]() . Both
. Both ![]() and
and ![]() have eight symmetry operations modulo lattice translations however the orthorhombic crystal system of
have eight symmetry operations modulo lattice translations however the orthorhombic crystal system of ![]() has higher symmetry than the monoclinic crystal system of
has higher symmetry than the monoclinic crystal system of ![]() .
.















Densest  and
and  Octahedral Molecule Packings
Octahedral Molecule Packings
Similar to the tetrahedral case, the close-packed space groups we have selected are also based on the densest ![]() octahedral molecule packing. Consequently, all densest packing configurations of octahedral molecules within these groups share the same sphere close-packing density of
octahedral molecule packing. Consequently, all densest packing configurations of octahedral molecules within these groups share the same sphere close-packing density of ![]() .
.















Densest  and
and  Cuboctahedral Molecule Packings
Cuboctahedral Molecule Packings
The computational experiments have confirmed our choice of symmetries for maximally dense tetrahedral and octahedral molecular packings. Therefore, the globally densest cuboctahedral molecular packing will be among the maximally dense packings in our list of closest-packed space groups.
Let’s have a look at the results from packing searches. We provide an algebraic expression for the configuration’s density alongside the numerical value in cases where we were able to determine one.


















Global Cuboctahedral Molecule Packing Maximiser
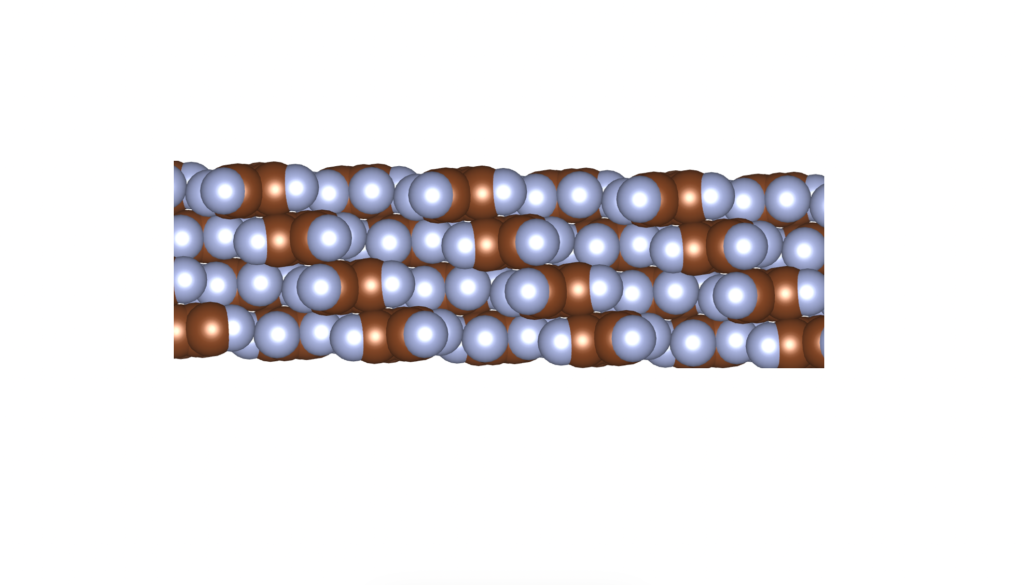
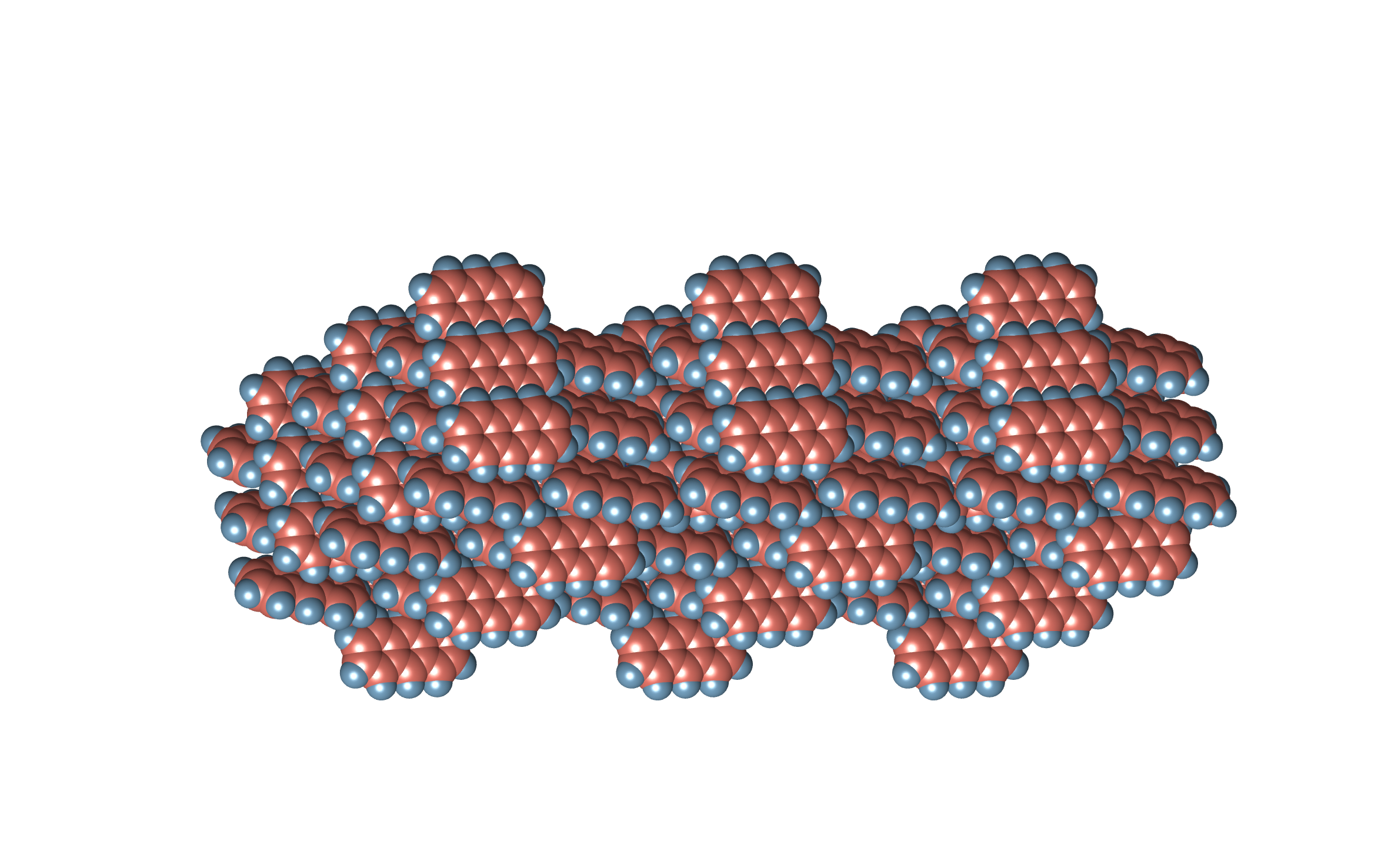
We have finally reached the conclusion of our investigation into the mystery we began with: is the GEOMAG cuboctahedral molecule packing model the global packing density maximum?
The answer is no. The structure in the animation represents the densest ![]() |
| ![]() packings, with a density of
packings, with a density of ![]() , which is the second densest cuboctahedral molecule packing in our list. Have a look at the comparison between the GEOMAG model and the structure from our computational experiment.
, which is the second densest cuboctahedral molecule packing in our list. Have a look at the comparison between the GEOMAG model and the structure from our computational experiment.


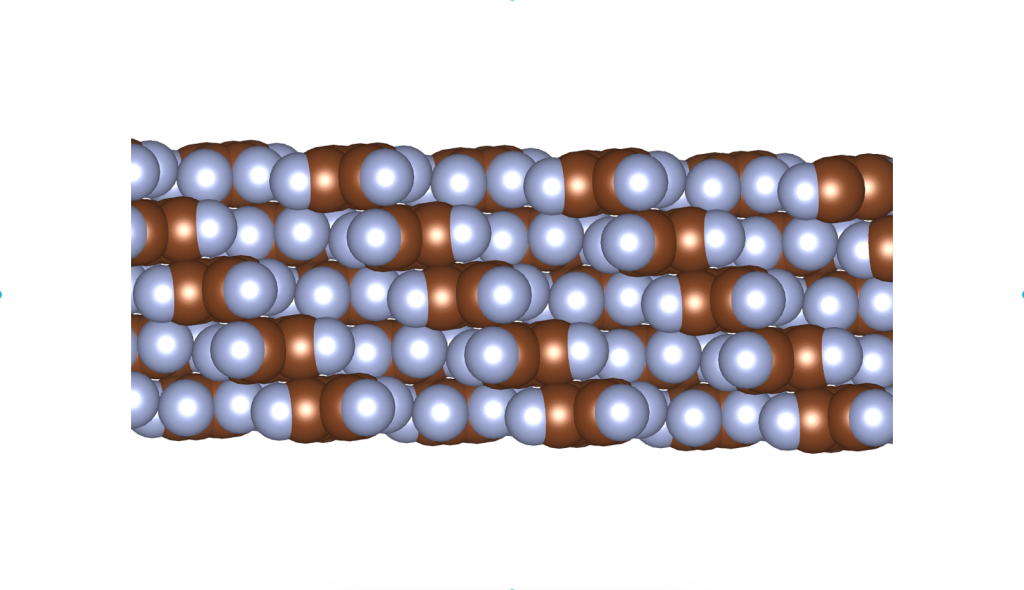
In contrast to the ![]() |
| ![]() configuration, the globally densest packing of cuboctahedral molecules is the
configuration, the globally densest packing of cuboctahedral molecules is the ![]() configuration, which achieves a packing density of
configuration, which achieves a packing density of ![]() . The GEOMAG model representing this arrangement appears like this:
. The GEOMAG model representing this arrangement appears like this:


How do we know it is the global maximum? Well, the ![]() packing density is just
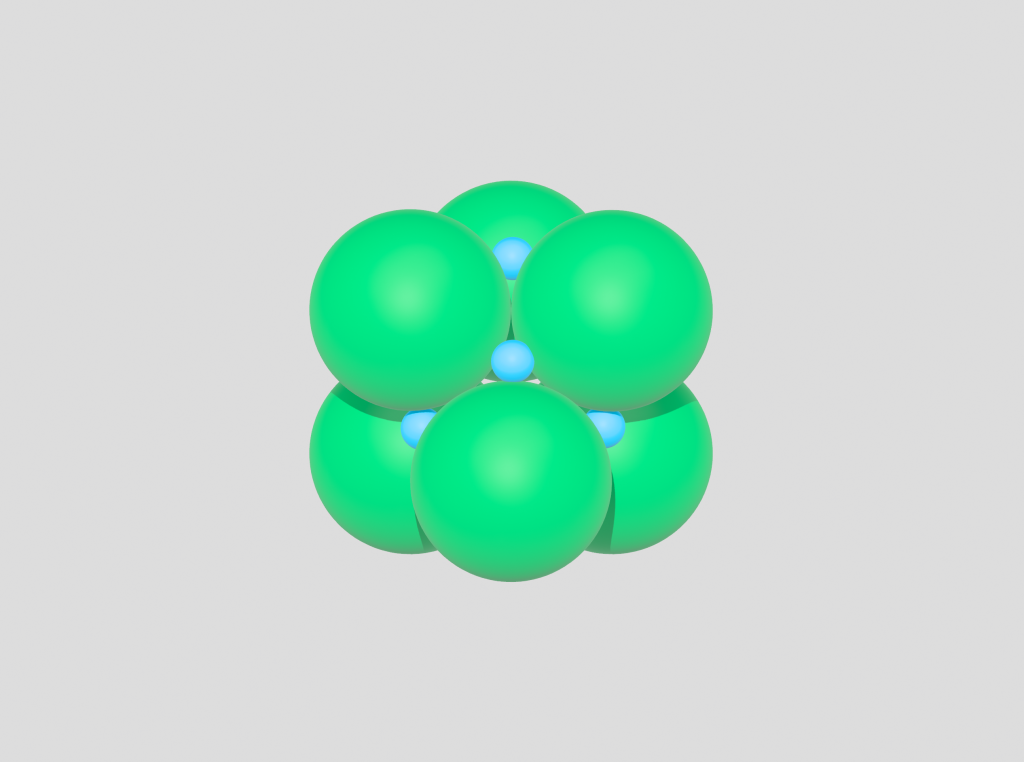
packing density is just ![]() short of the densest packing of equal spheres, and by construction our cuboctahedral molecule model is a collection of equal spheres with centres on the twelve vertices of a cuboctahedron, missing exactly one sphere on the inside. Conversely, the twelve spheres are in the densest possible arrangement around a single one—or shall we say—in the vector equilibrium of Buckminster Fuller.
short of the densest packing of equal spheres, and by construction our cuboctahedral molecule model is a collection of equal spheres with centres on the twelve vertices of a cuboctahedron, missing exactly one sphere on the inside. Conversely, the twelve spheres are in the densest possible arrangement around a single one—or shall we say—in the vector equilibrium of Buckminster Fuller.
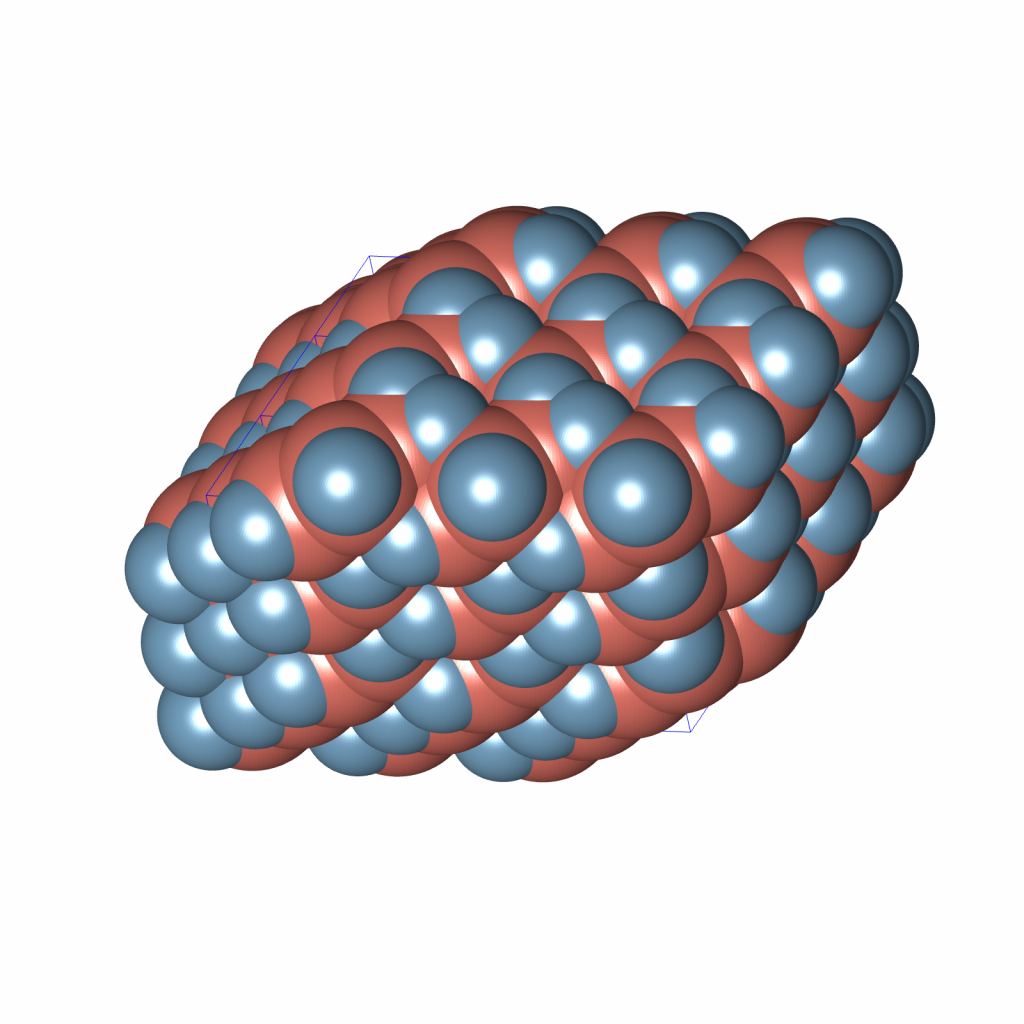
In fact, due to the radial symmetry of the cuboctahedron, the ![]() packing can be realised as a lattice packing — or, in H–M notation, as a
packing can be realised as a lattice packing — or, in H–M notation, as a ![]() packing — as shown in the image on the left below.
packing — as shown in the image on the left below.



The red and green spheres represent a complementary packing to the blue cuboctahedral module packing. The spheres missing at the centre of each cuboctahedron molecule represent the centres of mass of each cuboctahedron. Moreover, the arrangement of the red central spheres in the packing lies at the vertices of a rhombic dodecahedron – an edge is created whenever any two cuboctahedra come into contact.
What is the takeaway from examining the global packing maximum configuration? We observe two levels of locally maximal close packing:
1. Atomic – Locally maximal dense packings between atoms of different molecules, where each atom touches seven atoms from neighbouring molecules.
2. Molecular – Locally maximal dense packings between molecules, where each molecule touches 14 surrounding molecules, giving each molecule a coordination number of 14.
A practical consequence of these observations is that, in our molecular packing algorithm, when searching over space groups ![]() and
and ![]() (both triclinic crystal systems), we need to check for overlaps up to the second neighbouring unit cells. However, when searching over space groups with the monoclinic crystal system, it suffices to check overlaps only in the first neighbouring unit cells, which speeds up packing computations substantially.
(both triclinic crystal systems), we need to check for overlaps up to the second neighbouring unit cells. However, when searching over space groups with the monoclinic crystal system, it suffices to check overlaps only in the first neighbouring unit cells, which speeds up packing computations substantially.
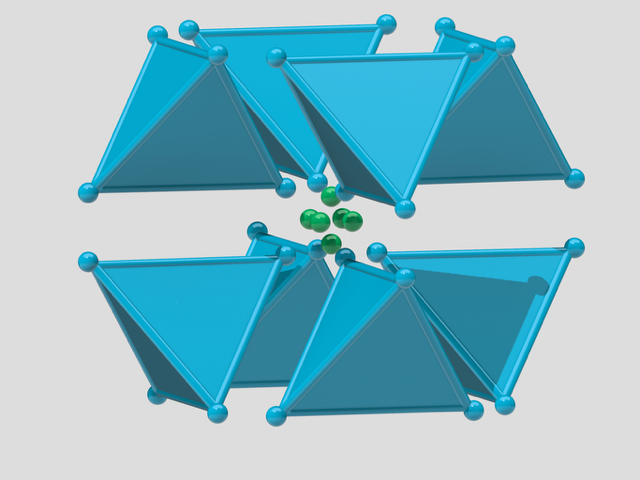
Incidentally, the rhombic dodecahedron is the cell of the rhombic dodecahedral honeycomb, which is the dual honeycomb to the tetrahedral–octahedral honeycomb with which we began our investigation. In the images below, you can see a visualisation of the rhombic dodecahedral honeycomb along a GEOMAG structure showcasing the relationship between tetrahedral, octahedral, and cuboctahedral molecular packings. Notice the inverted octet truss in the space frame generated by tetrahedral | octahedral molecular packings.


Compared to the tetrahedral and octahedral GEOMAG molecule models, the cuboctahedral molecule is not rigid. Since it is hollow on the inside (it is missing 12 bars that would otherwise stabilise the molecule model and make it rigid), it has multiple degrees of freedom of motion (see the GEOMAG Jitterbug transformation of the cuboctahedron into the icosahedron in Ionic Molecular Crystallisation Continued: Tetrahedra, Symmetry, and Salt-Like Molecular Architectures).
The interesting part of this property of the ![]() and
and ![]() GEOMAG models is that, in both configurations, the molecules do not collapse under the lattice forces. On the contrary, the lattice configuration structurally stabilises both models.
GEOMAG models is that, in both configurations, the molecules do not collapse under the lattice forces. On the contrary, the lattice configuration structurally stabilises both models.
A direct application of this observation in materials science could be a starting point for a geometric bias to guide inverse design search for novel porous materials, for instance, using a cuboctahedral molecular building block.
What Do the Space Groups  ,
,  ,
,  ,
,  ,
,  , and
, and  Have in Common?
Have in Common?
For one, they are relatively low-symmetry subgroups of the space group ![]() , which is the full symmetry of the face-centred cubic lattice.
, which is the full symmetry of the face-centred cubic lattice. ![]() has 48 elements modulo lattice translations, compared with a maximum of 8 in our closest-packed space groups.
has 48 elements modulo lattice translations, compared with a maximum of 8 in our closest-packed space groups.
These are also the six most frequent space groups in the Cambridge Structural Database as of 1 January 2025 (CSD Space Group Statistics), accounting for approximately 82.7% of the total 1,359,039 structures. If we leave out space group ![]() , the remaining groups are all centrosymmetric, comprising 75% of the CSD in total.
, the remaining groups are all centrosymmetric, comprising 75% of the CSD in total.
The truth is, we have been somewhat misleading. Thanks to A. I. Kitaigorodsky, we had a good estimate of the closest-packed space groups from the beginning. Below is a table from the book Molecular Crystals and Molecules by A. I. Kitaigorodsky, published in 1973, showing these.

However, as a result of our cuboctahedral molecule packing explorations, we can now add an additional column to the table. To paraphrase A. I. Kitaigorodsky: for molecules with tetrahedral symmetry ![]() , the closest packing is attainable in space group
, the closest packing is attainable in space group ![]() .
.
Acknowledgement
I am grateful to Henry Segerman for mentioning the orbifold plane group notation during our conversation at the ICERM Geometry of Materials workshop, and for drawing my attention to the book The Symmetries of Things by John H. Conway, Heidi Burgiel, and Chaim Goodman-Strauss. From now on, I am following William Thurston’s commandment:
Thou shalt know no geometrical group save by understanding its orbifold.








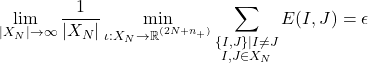
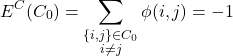
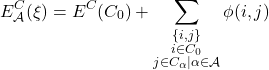







![Rendered by QuickLaTeX.com \begin{equation*} E_{LJ}=\sum_{\substack{\{I,J\} \\ I \cap J =0}} \sum_{\substack{(i,j ) \\ i \in I, j \in J}} \left[\left(\frac{1}{r_{(i,j)}}\right)^{12} - \left(\frac{1}{r_{(i,j)}}\right)^{6}\right] \end{equation*}](https://milotorda.net/wp-content/ql-cache/quicklatex.com-562681f5736399b7414a50ca69c95723_l3.png)
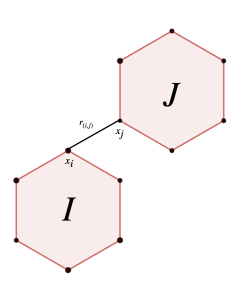
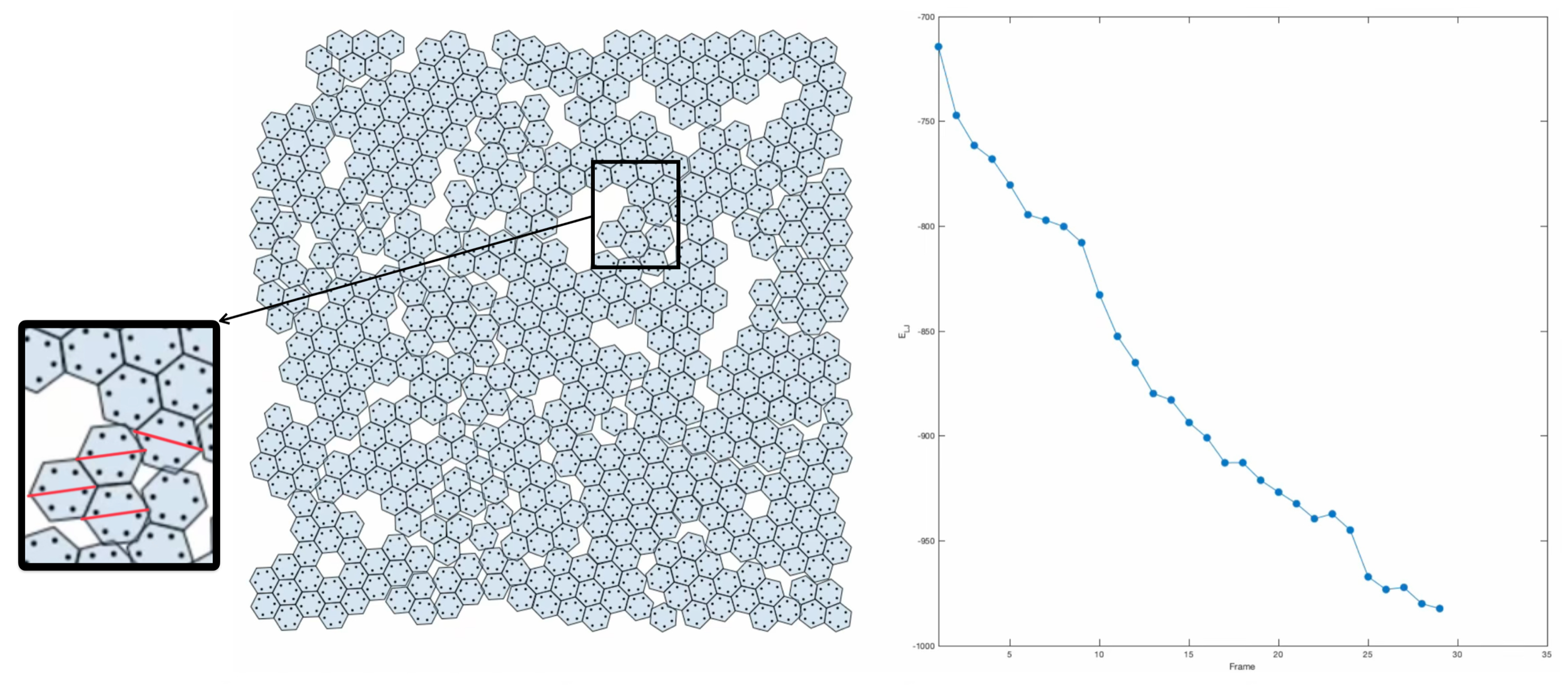
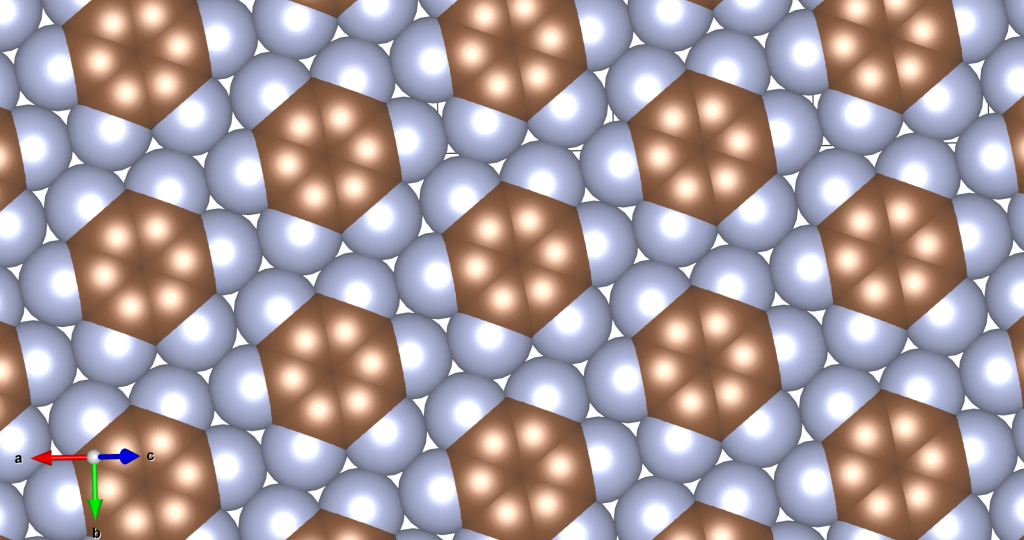

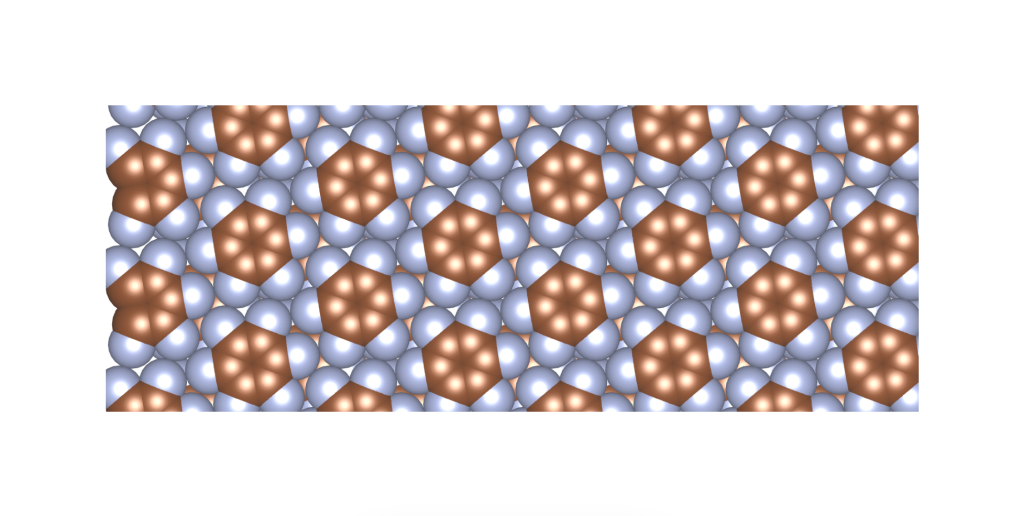




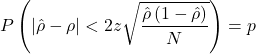


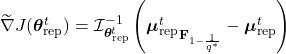 in the tangent space
in the tangent space